Electrometry

In real materials the magnetic degrees of freedom (spins) are often coupled to dielectric polarization (minute displacements of charged ions) via spin-orbital interactions. Due to the coupling through measurements of the dielectric response once can learn of magnetic quantities (e. g., spin-chirality) that are normally inaccessible experimentally.

Dielectric permittivity is particularly easy to measure with great accuracy. Just put your sample in a capacitor and measure the capacitance! Here is an example of a dielectric anomaly measured on the boundaries of the field-induced magnetic Bose-Einstein condensate in the frustrated ferro-antiferromagnet Rb₂Cu₂Mo₃O₁₂ (L. Huberich, S. Hayashida and D. Flavian - to be published).

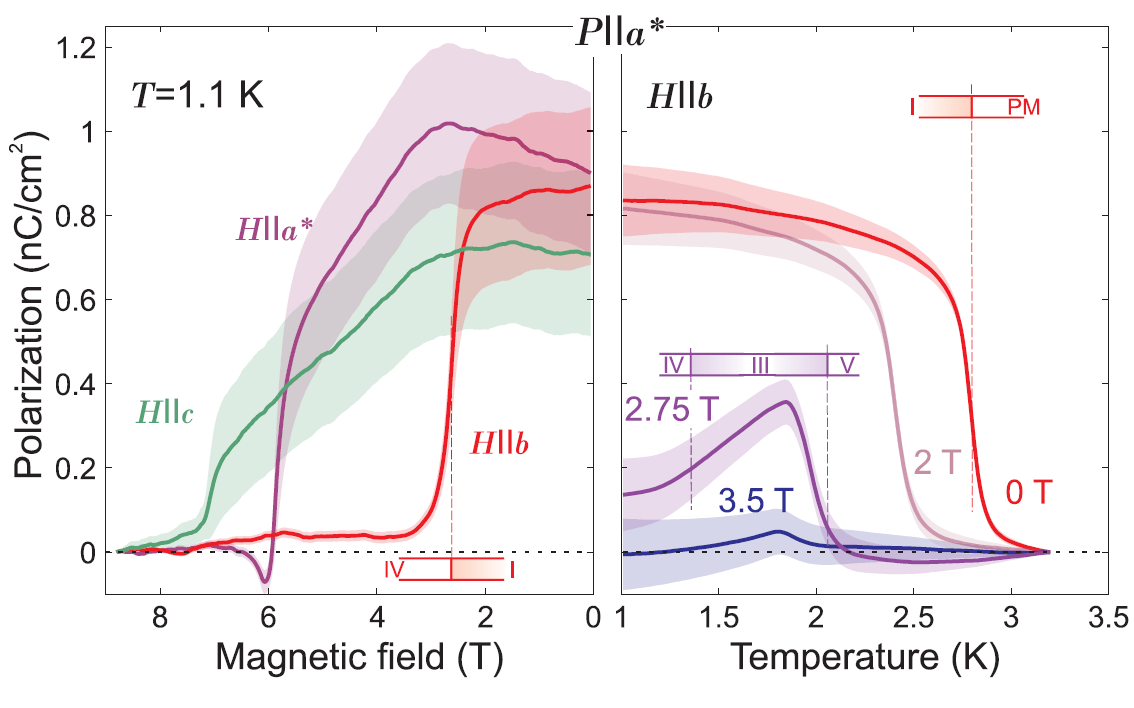
Electrical polarization is much harder to measure. One puts the sample in the same capacitor, but now one has to integrate the tiny (femto-Amperes!) pyroelectric current flowing into it. Here are some data illustrating this technique has been employed to study the complex ferroelectric phases of the frustrated quantum magnet linarite. For details see external page K. Yu. Povarov, Y. Feng, and A. Zheludev, Phys. Rev. B 94, 214409 (2016).

The dielectric response in the vicinity of magnetic quantum phase transitions may in certain cases be non-linear. This results in higher-order harmonic generation in AC measurements. Such data carry information on domain dynamics. This example shows the magnetic field evolution of the complex non-linear dielectric susceptibility up to the 6^th order measured in the frustrated "spin-tube" antiferromagnet Cu₂Cl₄⋅H₈C₄SO₂. For more details see external page K. Yu. Povarov, A. Reichert, E. Wulf, and A. Zheludev, Phys. Rev. B 92, 140410(R) (2015).